Surface magnetism of a magnet refers to the surface magnetic induction intensity of a magnet at a certain point, which can be divided into two situations:
1) Two magnets with the same shape and exactly the same size, the magnet with high apparent magnetism has strong magnetism.
2) Two magnets with different shapes or sizes cannot simply judge the magnetism of the magnets by the apparent magnetic height.
It should be stated here that surface magnetism is not equal to magnetism. Therefore, the higher the surface magnetism, the stronger the magnetic force is not necessarily, which is related to the size, performance and usage of the magnet.
Surface Magnetic Field of Neodymium Magnet
The surface magnetic field refers to the magnetic induction intensity at a certain point on the surface of the magnet, the unit is Gauss(Gs) or Tesla T (1T=10000Gs). The magnetic field of different positions on the surface of the magnet is not the same. Generally speaking, the “surface magnetic field” refers to the center of the working surface. surface magnetic field is the most easy to directly measure the performance parameters of the magnet in daily life. It can be directly measured with a Gauss meter, which is very convenient.
The Hall sensor elements on Gauss meters of different manufacturers are different, and the measured magnetic field of the same magnet is not exactly the same. The surface magnetic measurement is not objective and cannot fully reflect the parameters of the magnet performance, so it is not recommended to be used as an evaluation indicator for product transactions.
Calculation of Surface Magnetic Field of Neodymium Magnet
Since the sintered NdFeB magnet has a very high magnetic anisotropy field, the magnetization vector is arranged in the easy magnetization direction, so we can regard it as a uniform magnetization, so that the Biot–Savart law can be used to calculate the magnetization in space magnetic field. At present, many surface magnetic field calculation models on the Internet are based on this principle to derive the calculation formula, but there are two assumptions, one is that the magnet is a completely uniform magnetized body, the other is that the demagnetization curve is completely straight, and the actual situation is not completely true, so there is a certain difference between the calculated result and the actual measurement result. In addition to remanence, the surface magnetic field of a magnet is greatly affected by its shape and size, and the applicable surface magnetic field calculation formulas for magnets of different shapes are different.
Rectangular Shape
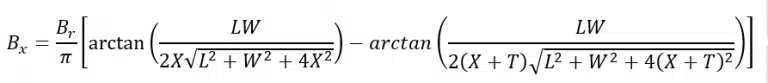
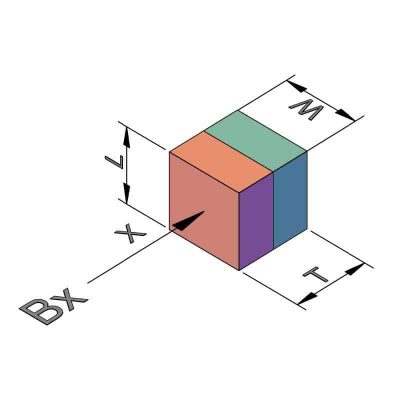
Magnet Grades
Remanence (Br)
Gauss
Length (L)
mm
Width (W)
mm
Thickness (T)
mm
strength
Gauss
Cylinder Shape
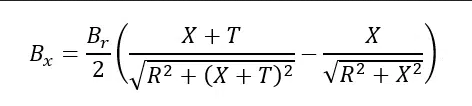
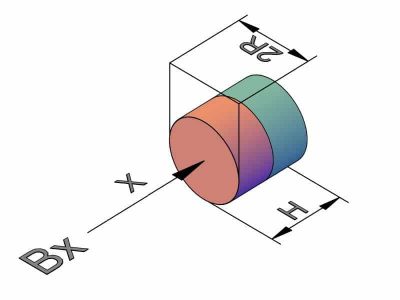
Magnet Grades
Remanence (Br)
Gauss
Diameter (D)
mm
Thickness (H)
mm
Strength
Gauss





